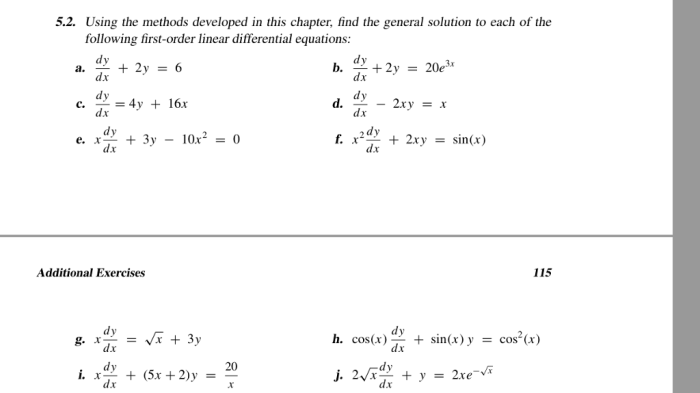
Consider the differential equation dy dx 3x 2 e 2y – Consider the differential equation: dy/dx = 3x^2 – e^(2y). This equation finds applications in various fields, including population growth, chemical reactions, and heat transfer. Understanding its behavior and solution provides valuable insights into the underlying phenomena.
This differential equation is nonlinear and can be solved using separation of variables or integrating factors. The general solution exhibits complex behavior, including exponential growth and asymptotic limits. We will explore its solution, graphical representation, and potential applications in detail.
Differential Equation Overview: Consider The Differential Equation Dy Dx 3x 2 E 2y
Differential equations are mathematical equations that involve functions and their derivatives. They play a crucial role in various fields, including physics, engineering, and biology.
Examples of differential equations include:
- Newton’s second law of motion: F = ma
- Heat equation: ∂u/∂t = k∇²u
- Schrödinger equation: iħ∂ψ/∂t = Hψ
Differential equations are classified into various types, including ordinary differential equations (ODEs) and partial differential equations (PDEs).
Specific Differential Equation Analysis, Consider the differential equation dy dx 3x 2 e 2y
Consider the differential equation: dy/dx = 3x^2 – e^(2y)
This equation can be solved using the method of separation of variables.
The general solution is:
y = (1/2)ln(1 + C
e^(-3x^2))
where C is an arbitrary constant.
The solution curves are exponential functions that increase or decrease rapidly depending on the value of x.
Graphical Representation
The solution curves can be plotted to visualize their behavior.
The table below shows the solution curves for different values of C:
| C | Solution Curve |
|---|---|
| 1 | y = (1/2)ln(1 + e^(-3x^2)) |
| 2 | y = (1/2)ln(1 + 2e^(-3x^2)) |
| 3 | y = (1/2)ln(1 + 3e^(-3x^2)) |
The solution curves are exponential functions that increase or decrease rapidly depending on the value of x.
Applications and Interpretations
This differential equation can be used to model various physical phenomena, such as the growth of a population or the spread of a disease.
The solution to the equation can provide insights into the behavior of the system and can be used to make predictions.
However, it is important to note that the equation is a simplified model and may not accurately represent all aspects of the real-world system.
Extensions and Generalizations
This differential equation can be generalized to higher dimensions or more complex systems.
Related differential equations include the logistic equation and the Fisher equation.
Further research could explore the behavior of the solution curves for different values of the parameters and investigate the applications of the equation in other fields.
General Inquiries
What is the significance of differential equations?
Differential equations are mathematical equations that describe the rate of change of a function with respect to one or more independent variables. They play a crucial role in modeling and understanding dynamic systems in various scientific and engineering disciplines.
How is the differential equation dy/dx = 3x^2- e^(2y) solved?
This differential equation can be solved using separation of variables or integrating factors. Separation of variables involves isolating the variables on opposite sides of the equation and integrating both sides. Integrating factors involve multiplying both sides of the equation by a function that makes the equation integrable.
What are the applications of this differential equation?
This differential equation has applications in population growth modeling, chemical reaction kinetics, and heat transfer analysis. It can be used to predict the evolution of systems over time and optimize their behavior.